
We have already used circles extensively to create various grids for a number of patterns. In this lesson we are using circles for their own sake, namely in two types of constructions: spirals and inscribed circles.
Spirals
Spirals come in several different types. The distance between turnings, and the angle of each turning, determines their appearance. Some can be defined using a mathematical equation, which translates, for specific spirals, into easy geometric constructions—approximate, but quite good enough for the eye.
Regular or Archimedean Spiral
This spiral is defined by an equal distance between turnings, so that it has a concentric appearance. It is drawn by moving the compass point from one point to the other in a base figure that can be a segment (two points), a triangle, a square, etc. The more points, the tighter and more perfect the spiral, but as that also makes construction more tedious, a hexagon is the highest one usually goes.
Spiral Built on Two Points
Step 1
On a horizontal line, draw a semicircle that's as small as possible. This is the first turning of the spiral, and the two points where it cuts the line are the construction points.

Step 2
Place the compass on one of the points, open it to meet the other, and draw a semicircle on the other side of the line. The two semicircles make a continuous curve.

Step 3
Move the compass back to the first point, open it to meet the end of the curve, and draw another semicircle.

Step 4
Continue in this vein, moving the compass from one of the construction points to the other and adjusting the opening each time to take up the curves where you left off.


Carry on as much as desired. The spiral will look like this:

Spiral Built on Three Points
The method is the same but we start with an equilateral triangle, the sides of which are extended. The compass will be moving from point 1 to 2 to 3 then back to 1, and so on. If the sides are extended as shown here, the spiral turns clockwise (and the compass moves from point to point in a clockwise direction).

Step 1
Draw the first arc.

Step 2
Move to the next point, adjust the opening and draw the next arc.

Step 3
Move to the third point and repeat.

After a few turnings, the spiral looks like this:
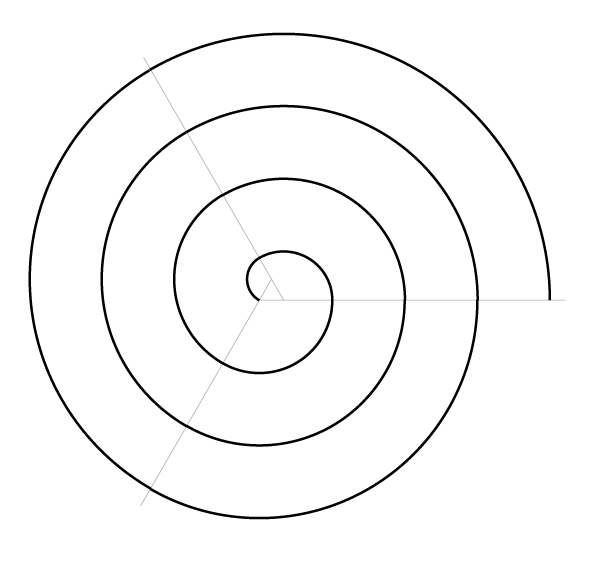
Spiral Built on Four Points
Our base is now a square, and we are still working clockwise. As the angle of the turnings becomes smaller (first it was 180º for each, then 120º, now 90º), the spiral becomes smoother.
Step 1
Draw the first quarter-circle.

Step 2
Move to the second point, adjust the compass opening and draw the next quarter-circle.

Step 3
Repeat with the third and fourth points.


Step 4
How the spiral looks after a few turns:

Spiral Built on Six Points
With a hexagon as base, the construction is really the same. The critical part is drawing the bases and the extension of their sides very accurately. Then just run through the six points:






The spiral after a few turns:

When these spirals are placed side-by-side, we can appreciate how much smoother and more perfectly circular they are when the base has a higher number of points.

Golden Spiral
In contrast to the regular spirals above, the distance between successive turnings inlogarithmic spirals grows in a geometric sequence. Such spirals, found in the growth of many organisms, are self-similar: the size of the spiral increases but its shape is not altered (for this it was also named spira mirabilis, the "miraculous spiral"). The golden spiral is a type of logarithmic spiral with a growth factor linked to the Golden Number.
The simplest way to draw such a spiral is to start from its outer boundaries, contrary to the previous one. We'll therefore start by constructing a golden rectangle (I'll explain what it is when that's done.)
Step 1
Construct a square. (Forgotten how? See Working With 4 and 8.)

Step 2
Extend the sides AB and DC.

Step 3
With the dry point on E and the compass open to EC, draw an arc that cuts the extended AB at G.

Step 4
Move the dry point to F and draw an arc that cuts the extended CD at H.

Step 5
Join GH to complete the rectangle.

This is called a golden rectangle because AB/AG = BG/AB, in other words the relation of the longer side to the whole segment is the same as that of the shorter side to the longer.
An A4 piece of paper (or any other size in the A series) is a golden rectangle, so you could use its total surface as the outer rectangle, and go straight to step 6.
Step 6
We now need to break this rectangle down into squares. We already have the first square. The next one will be taken out of the rectangle BGHC.
Place your dry point on B and open it to the length of the short segment. Mark I on BC.

Move the dry point to G and mark J on GH.

Step 7
Connect IJ: we now have a square BGJI, and a new rectangle left over.

Step 8
Repeat this operation in each successive rectangle, always creating the square against the outer edge of the rectangle.

When we have enough squares, or they become too small to work with, we can draw the spiral proper.
Step 9
Place the dry point on C, let the opening be equal to the side of the first square, and draw a quarter of a circle DB.

Step 10
Move the dry point to I, reduce the opening to the side of the second square, and draw an arc BJ.

And so on through all the squares...




The feel of this spiral is very different from the concentric and even static appearance of the regular spirals: it's much less contained, with dynamic movement.
Inscribed Circles
Circles can be inscribed, i.e drawn inside a shape in such a way as to be tangent to its sides, in angles, polygons or other circles. This device is the basis for much of the decorative geometry of the West, for instance in Celtic illumination or Gothic rose windows. We'll look at two basic constructions that we can use with any polygon or any number of circles inside a circle, and then construct two full-fledged windows with their tracery.
Circle in a Sector
This method allows you to fit the number of circles of your choice inside a circle. Start by dividing your circle evenly in the desired number of sections, then for each sector proceed as follows. The sector shown here is from a circle divided in six.
Step 1
Bisect the sector. The bisector cuts the arc at Q.

Step 2
We now need to draw the perpendicular to PQ in Q. With the dry point of the compass on Q, and any opening, draw an arc that cuts the bisector at point A.

Step 3
Move the dry point to A and draw another arc cutting the first at B.

Step 4
Connect the line AB and extend it somewhat.

Step 5
With the same compass opening and the point on B, mark point C.

Step 6
CQ is the perpendicular to PQ.

Step 7
Extend one side of the sector to cut CQ at point E.

Step 8
Bisect the angle QEP.


This bisector cuts QP at a point O.

Step 9
Point O is the centre of the circle inscribed in this sector. The circle can now be drawn, with the compass point on O and the opening set to OQ.

Here are some possibilities, depending on the number of sectors the circle was divided into. Note that, the circles being tangent, the arcs between their contact points can be omitted to create rosettes.

Circle in a Kite
This method is to fit a number of circles in a polygon equal to the number of sides of that polygon (three circles in a triangle, five in a pentagon, four or eight in an octagon...).
First connect the centre of each side to the centre of the polygon, thus dividing the polygon into kites, and then proceed as follows for each kite.

Step 1
Bisect ACB. This bisector cuts AB at O.

O is the centre of our inscribed circle, but in order to determine the radius of the circle accurately, we need to find a point F on AD so that OF is perpendicular to AD. This is the purpose of the remaining steps:
Step 2
With the dry point on A and compass open to AO, draw an arc.

Step 3
Move the dry point to D and repeat, to find point E.

Step 4
Join OE to cut AD at F.

Step 5
The inscribed circle can now be drawn, with centre O and radius OF.

As with the previous construction, different polygons will result in different shapes, and the the inner arcs can be erased to create rosettes.

Triskele Window (Three Circles)
Such church windows betraying a Celtic influence can be spotted in many places around the British Isles.
Step 1
Start with a circle. Divide it into six and draw the diameters.

Step 2
Join three of these points to create an equilateral triangle.

Step 3
With the compass opening below, draw the circle inscribed in the triangle.

Step 4
Draw another triangle, inscribed in this circle.

Step 5
With the compass opening below, draw the three circles centered on the points of the triangle.


Step 6
With the compass opening below, draw the circle in which the three smaller ones are inscribed.

If you just want a linear rendering, you can stop here and ink the following arcs:

To draw the tracery of the window, i.e to give these lines their own thickness and detailing, (where the "line", being the window frame, has thickness and detailing of its own), carry on...
Step 7
Place the dry point where one of the intersection of a diameter with the last circle we drew, and set the opening to the difference between the two large circles. Draw a small circle.

Step 8
Return the dry point to the original centre and open it as shown. Draw a third, innermost large circle.

Step 9
Now, for each of the three circles, draw an inner circle using the opening shown below.


Step 10
Now change the opening as shown, and for each of the three, draw this arc:


Step 11
You can now ink the two outer circles...

... then the inner drop-shapes...

... and finally the central lines of the triskele.


Rosette Window (Eight Circles)
This is a window from the West front of Chartres cathedral, and the oldest in the building.
Step 1
Start with a large circle. Divide it in eight, by following the steps for drawing a square (there's no need to draw the square itself, because we only need its diagonals).


Step 2
Bisect half of the sectors to divide the circle further into 16.


There are now eight diameters. Number the points for clarity.

Step 3
Join the even-numbered points to create a static octagon.

Step 4
The sides of the octagon cut the diameters at eight points. Join these to create an inscribed, dynamic octagon.

Step 5
Now draw one more static octagon inscribed in the previous one.

Step 6
Now, returning to the numbered points, join the following pairs: 2-8 and 10-16, then 4-14 and 6-12.

Step 7
Join 2-12 and 4-10, and finally 6-16 and 8-14.

Notice the following places where three lines intersect: they are the centres of the eight circles forming the rosette.

Step 8
With the compass opening below, draw a circle centered on each of these points.


Ink the arcs shown here.

Step 9
Change the opening of the compass as shown here, and repeat. There is no need to draw the full circles—you can stop the arcs where they meet a diameter, and ink them that way.


Step 10
Change the compass opening once more and repeat, again stopping at diameters.


Step 11
Join the open ends of the arcs.

Step 12
Ink the lines between arcs; they are portions of the diameters.

Step 13
With one last compass adjustment, draw and ink the circle below.

Step 14
Finally, ink the outer circle.


With this chapter on circles, we have completed the basic part of these lessons on geometric designs. From next month on we will focus on complete patterns and motifs of increasing complexity, from both East and West.







